Infrared Spectrum

There are many different forms of radiant energy for eample, ultraviolet, visible, and infrared light. Each form of light differs only in its wavelength or frequency. Infrared radiation has relatively long wavelengths (2 microns) and frequencies (2000 cm^-1). It is the type of radiation that is given off by embers in a fire and which you sense as warmth. On a microscopic scale infrared radiations is associated with molecular vibrations. We will be concerned primarily with the middle infrared region with frequencies from about 300 cm^-1 to 3000 cm^-1.

Molecular Vibrations

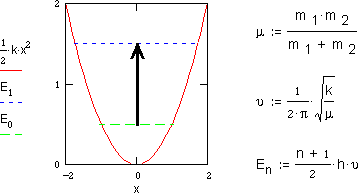
As the picture at the top of this page shows, atoms in a molecule do not maintain fixed positions but acutally vibrate back and forth about an average bond distances. Since this motion is microscopic it is quantized into descrete energy states by the laws of quantum mechanics. These laws indicate that for the simple stretching motion of a diatomic molecule the vibrational frequency depends on the stiffness of the chemical bond as determined by its force constant k and on the mass of the atoms as determined by the reduced mass .

Stiff double bonds vibrate faster than loose single bonds. and heavy atoms will vibrate slower than light atoms. For this reason organic functional groups that have characteristic masses and bond strengths fall into particular regions in the infrared spectrum. For example, hydrogen stretching frequencies occur at large frequencies of about 3000 cm^-1. Stretching frequencies in inorganic complexes on the other hand occur at low frequencies because the atoms are heavy and weakly bonded. For example, Mo-Cl bond stretching occurs in the far infrared at about 250 cm^-1.

At room temperature most of the molecules will be in their lowest vibrational energy state or zero-point energy E₀. Absorption of light with the approriate energy allows the molecule to become "excited" into the next higher vibrational energy state E₁. Such absorption of infrared light can only occur, if the dipole moment of the molecule is different in the two vibrational states. This changing dipole results in a molecular oscillating electric field. Since light itself is a oscillating electric field it can use the oscillating molecular field as a electronic handle to grab and shake the molecule into a higher vibrational state. The requirement that the absorption of a vibrational quantum be accompanied by a change in diople moment is an example of a spectroscopic selection rule. Vibrational transitions that obey this rule are said to be infrared active. Vibrational transitions that do not are said to infrared-inactive. For example, carbon monoxide and hydrogen chloride absord infrared light, but diatomic hydrogen or oxygen do not.

Normal Modes of Vibration

More complex polyatomic molecules have more possible vibrations. A nonlinear molecule,like methylene dichloride (CH2Cl2), containing N atoms can have 3N-6 fundamental or normal modes of vibration. As a result, the infrared spectrum of organic molecules are rather complex. Methylene dicloride would have 9 different normal modes of vibration. The complex spectrum of a Polyatomic organic compound has two distinct molecular vibrations, stretching and bending. Vibrations of bonds involving hydrogens are especially important because atoms of low mass vibrate faster. The spectrum of n-octane, only has four major asposortion bands at 2925, 1465, 1380, and 720 cm-1. We can understand the spectrum of n-octane by considering the folowing solution specturm and Spartan simulation of methylene dichloride.

Spartan Simulation

The nine normal modes of methylene dichloride are shown in the following table. Only the A₁, B₁, and B₂ are infrared active. You can change the view and rotate the molecule by puting the curson into the molecle window and holding the right mouse key down to bring up a CHIME window. Select Display/Spacefill/Van der Waals Radii. Details of the calculation can be found in Spartan output.

Going back to n-octane the four bands correspond to the C-H streching vibrations (2925 cm^-1), the CH2 and CH3 sissoring mode (1465 cm^-1), the CH3 rocking mode (1380 cm^-1), and the CH2 rocking mode (720 cm^-1).

S.F. Sontum
Created 8/97
Updated 8/01